Linear Programming Problem (LPP) Formulation with Numericals
Watch videoTo formulate Linear Programming Problem (LPP) for given statement type problems(numerical) is easy if we go through its Mathematical Model.
General Mathematical Modelling of LP
As explained in the video, mathematical model of LP consists of the following:
i) Objective Function (denote with “Z”)
ii) Decision variables (represented in terms of “x”)
iii) Constraints
Note: To know more about above terms you can go through the notes of the Basics of Linear programming, link to the notes- click here
Now, let us generate the mathematical model of the LPP:
Let,
Decision variables be with total ‘m’ constraints.
Now, LP can be formulate as:
Thus, we will have any of the three conditions applied on constraints as per the provided problem .
Steps for LPP Formulation
-
Identify the decision variables:
It is the most important step on LPP Formulation, because based on the decision variables only, the whole problem governs. We will learn about, how to identify decision variables from given numerical/problem in this note only. (Also you can go through video for the same. Link provided in cover page) -
Identify Objective Function (Z) and express it as a linear function of decision variables:
As we have seen above in mathematical model, objective of any problem can be maximize or minimize. Based on that, we will have to define the objective function in terms of decision variables. (For eg: Maximize )
-
Identify all constraints and express it as linear inequalities or equalities in terms of decision variables:
As we know that, in the real world all the resources are limited and so in each particular problem you will find some limitation/constraint on the use of available resources. -
Express decision variables as Feasible variables:
It means that, we have lastly define all the decision variables are greater than or equal to zero (For eg: )
Let’s move to numerical now,


Numerical 1: Two products ‘A’ and ‘B’ are to be manufactured. Single unit of ‘A’ requires 2.4 minutes of punch press time and 5 minutes of assembly time, while single unit of ‘B’ requires 3 minutes of punch press time and 2.5 minutes of welding time. The capacity of punch press department, assembly department and welding department are 1200 min/week, 800 min/week and 600 min/week respectively. The profit from ‘A’ is ₹60 and from ‘B’ is ₹70 per unit. Formulate LPP such that, profit is maximized.
Solution:
Tip: Always relate/treat LPP with real life problems for better understanding and ease of solution
Step 1: Identify the decision variables
Here, the decision has to be taken for how much quantities of product ‘A’ and ‘B’ to be manufactured in order to maximize the profit. (Quantities of product is governing this problem)
Let,
Quantity of product ‘A’ manufactured =
Quantity of product ‘B’ manufactured =
Step 2: Identify Objective Function (Z)
Here, the objective is to maximize the profit from product ‘A’ and ‘B’. Each unit produced product ‘A’ gives profit of ₹60, while ‘B’ gives ₹70. So, the objective function can be defined in terms of decision variables as:
Step 3: Identify all the constraints
Product ‘A’ manufactured by: Punch press and Assembly
Product ‘B’ manufactured by: Punch press and Welding
So, we have a total of three processes for the manufacturing of two products A and B.
Thus, there are total three constraints.
Now, we will bifurcate this problem based on processes to get a clear idea of constraints on resources, as follows:
Product ‘A’ requires 2.4 min and product ‘B’ requires 3 min of Punch press time.
Also,
punch press time is limited to 1200 min/week.
Product ‘A’ requires 5 min of Assembly time.
Also, assembly time is limited to 800 min/week.
Product ‘B’ requires 2.5 min of Welding time.
Also, welding time is limited to 600 min/week.
Step 4: Express decision variables as feasible variables
We assume that the decision variables has feasible solution, that implies - decision variables are greater than or equal to zero.
So, complete LP can be written as,
Note that, here the solution provided (in above numerical) to you with steps is just for explanation. You can always skip this step wise solution to LP problems and can use direct method as provided in video of this topic (click here to watch video).
Numerical 2: A tailor prepares two kinds of dresses. First kind of dress is having raw material cost of ₹150 and labour cost of ₹80, while the second kind of dress is raw material cost of ₹250 and labour cost of ₹100. He can sell the dresses of first and second kind at the rate of ₹300 and ₹500 respectively. First kind of dress takes 2 hours and second kind takes 2.5 hours of cutting. The total labour hours are restricted to 84 hours/week. Also, first kind of dress takes 1.5 hours of stitching and second requires 2 hours of stitching. The stitching hours are restricted to 60 hours/week. Formulate LPP, such that maximum profit can be earned by tailor.
Solution:
The solution of this numerical will be such that, you can present this in your examination. This will save your time and gives complete idea of your explanation too in examination. All the explanation stuff will be provided in {"Explanation"} brackets for your understanding only, in the solution of this problem.
Let,
Number of first kind of dress = units
Number of first kind of dress = units
{The decision variables selected here are the units of dresses of both kind so produced, as we are going to maximize the profit of tailor. Also, by understanding the whole problem you can easily make out that, to increase the profit, tailor needs to produce more goods.}
Now, Net Profit earned by selling a unit of first kind of dress
Net Profit earned by selling a unit of first kind of dress
{As you can understand that the problem provided here is based on revenue so generated by selling different kinds of dresses. Now, revenue will be the profit generated after excluding the raw material and manufacturing cost on each of these dresses. So, for example you can see above we have excluded raw material cost of first kind of dress ₹150 and labour cost ₹80 from total selling cost of ₹300.
Thus revenue/net profit generated by selling each unit of first kind of dress is ₹ (300-150-80) = ₹70 and the same for the second kind of dress.}
∴ The objective function can be defined as;
Now, constraints are provided on labour hours for cutting and stitching. Thus, LP is subject to the following constraints;
{As we can find from problem provided, the cutting and stitching hour requirements for both kinds of dresses. Also total hours are restricted on cutting and stitching is 84 hours/week and 60 hours/week respectively.}
So, complete LP can be written as,
Suggested Notes:
Modified Distribution Method (MODI) | Transportation Problem | Transportation Model
Stepping Stone | Transportation Problem | Transportation Model
Vogel’s Approximation Method (VAM) | Method to Solve Transportation Problem | Transportation Model
Transportation Model - Introduction
North West Corner Method | Method to Solve Transportation Problem | Transportation Model
Least Cost Method | Method to Solve Transportation Problem | Transportation Model
Tie in selecting row and column (Vogel's Approximation Method - VAM) | Numerical | Solving Transportation Problem | Transportation Model
Assignment Model | Linear Programming Problem (LPP) | Introduction
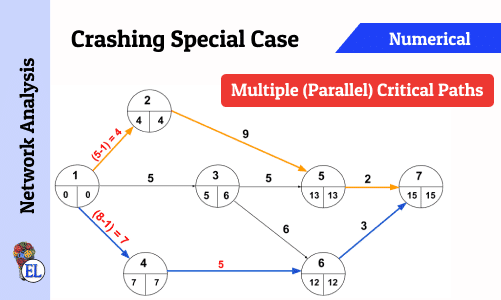
Crashing Special Case - Multiple (Parallel) Critical Paths
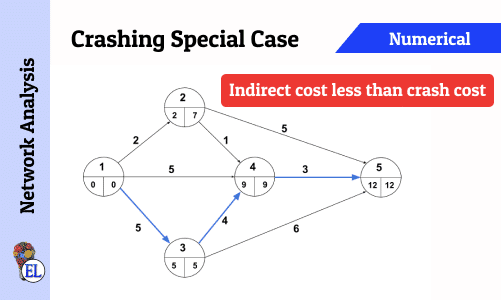
Crashing Special Case - Indirect cost less than Crash Cost
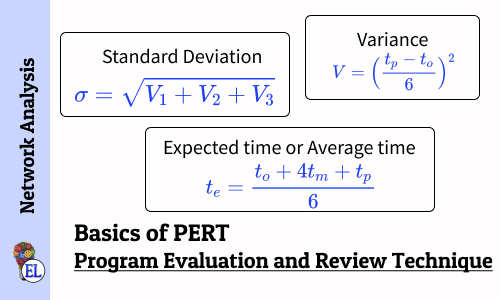
Basics of Program Evaluation and Review Technique (PERT)
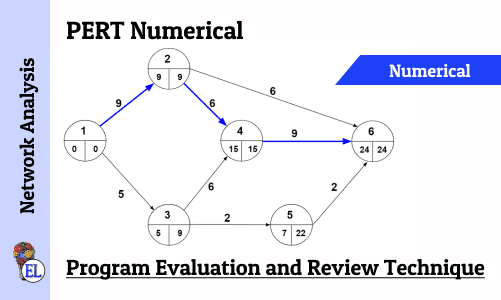
Numerical on PERT (Program Evaluation and Review Technique)
Network Analysis - Dealing with Network Construction Basics
Construct a project network with predecessor relationship | Operation Research | Numerical
Graphical Method | Methods to solve LPP | Linear Programming
Basics of Linear Programming

Comments:
All comments that you add will await moderation. We'll publish all comments that are topic related, and adhere to our Code of Conduct.
Want to tell us something privately? Contact Us
Post comment