Crashing Special Case - Indirect cost less than Crash Cost
Watch videoAs we have understand the method of crashing in the video, let us move to the special case of Crashing. (If you have not watched the video yet, click on the button above and clear your doubts about the method now.)
In this case of crashing we will have indirect cost incurred in the project less than the minimum cost slope of the critical activity.
We will discuss here how to solve such problem here! And also, learn how to obtain the minimum project duration and optimum minimum cost.
Also, we will understand the whole concept by solving the numerical and comparing it with practical-real life problem to get clear idea.
Let us start by considering the following numerical;
Numerical:
Table below shows the normal duration & cost and crash duration & cost of the various activities in a project. Find the optimum duration and minimum project cost, assuming the indirect cost of the project as Rs. 300/day.
| Activity | Normal Time(days) | Normal Cost(Rs.) | Crash Time(days) | Crash Cost(Rs.) |
|---|---|---|---|---|
| 1-2 | 2 | 800 | 1 | 1400 |
| 1-3 | 5 | 1000 | 2 | 2000 |
| 1-4 | 5 | 1000 | 3 | 18000 |
| 2-4 | 1 | 500 | 1 | 500 |
| 2-5 | 5 | 1500 | 3 | 2100 |
| 3-4 | 4 | 2000 | 3 | 3000 |
| 3-5 | 6 | 1200 | 4 | 1600 |
| 4-5 | 3 | 900 | 2 | 1600 |
Solution:
Let us solve this numerical, starting with Critical Path method (CPM) to obtain project completion time.
If you don't know about Critical Path method (CPM), then check out - CPM Video.
Network Diagram:
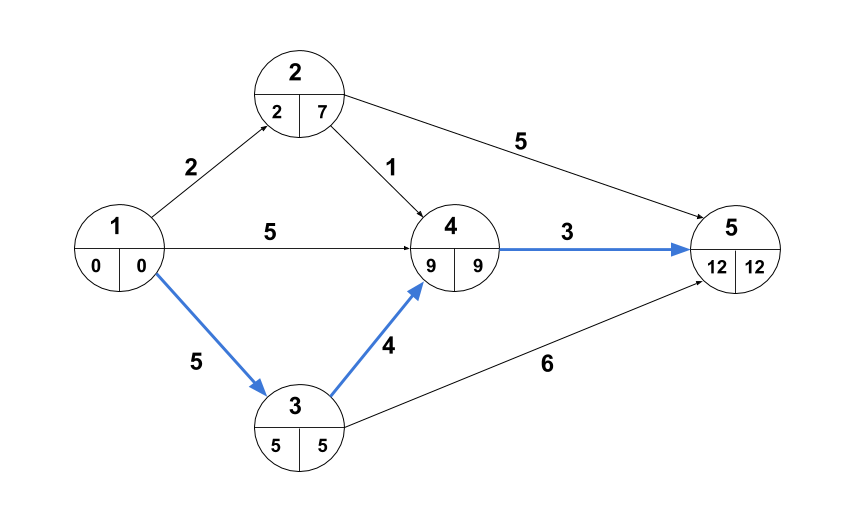 Network Diagram using CPM
Network Diagram using CPM
We can derive project completion time and critical path from above network diagram.
Also, we will derive project completion cost from the given data and network diagram.
If you don't know above mentioned terms like - Project Cost, Normal Cost, Crash Cost, Indirect Cost, and also don't know the calculation, then check out - Crashing video.
Now, let us begin with crashing method by finding cost slope from the given data as follows:
In examination you can only copy acivites in the first column and add second column for the cost slope. For better understanding, we are providing whole table again with the addition of the last column of cost slope. Same type examination tactics are always illustrating in videos provided by Education Lessons.
| Activity | Normal Time(days) | Normal Cost(Rs.) | Crash Time(days) | Crash Cost(Rs.) | Cost Slope = |
|---|---|---|---|---|---|
| 1-2 | 2 | 800 | 1 | 1400 | 600 |
| 1-3 | 5 | 1000 | 2 | 2000 | 333.33 |
| 1-4 | 5 | 1000 | 3 | 18000 | 8500 |
| 2-4 | 1 | 500 | 1 | 500 | Not possible |
| 2-5 | 5 | 1500 | 3 | 2100 | 300 |
| 3-4 | 4 | 2000 | 3 | 3000 | 1000 |
| 3-5 | 6 | 1200 | 4 | 1600 | 200 |
| 4-5 | 3 | 900 | 2 | 1600 | 700 |
Here, in the above table, activites which are generating critical path are highlighted with bold format.
Let us now identify the activity having minimum cost slope from the above highlighted activites.
We found that, acitivity 1-3 is having the minimum cost slope of 333.33.
Cost Slope is the minimum cost one has to pay, if crash any particular acitivity by 1 day.
But wait, we have given indirect cost is Rs. 300/day. So, if we move to step-by-step carshing for that activity, and reduce 1 day (suppose), then also, we will have new cost of project as follows:
Step-by-step crashing:
It means that we have to crash the acitivity in parts. Suppose as we have given in this numerical, for activity 1-3 normal time as 5 days, whereas crash time is 2 days. So, we can crash this activity 3 times in parts by crashing for 1 day at a time. Also, if you crash this activity 3 times, then remaining days for activity completion will be equal to crash time, and so you will not able to crash that activity further.
We are using step-by-step crashing to understand this miscellaneous numerical very easily. You can use direct method as well, as shown in the Crashing video. After completion of this numerical, your mind will be trained to identify such kind of numerical.


New project time & cost if crashed 1-3 by 1 day:
OR
So, we can observe that from above calculations, that the after crashing the critical acitivity, with minimum cost slope and by just 1 day (i.e., with least possible parameters); the total project cost will be increased only.
But, by crashing, our main aim is to reduce the project time with minimum cost. However, in this numerical if you crash with minimum parameters required for the crashing, then also project cost will be increased with reduction in duration of the project, and which is not profitable.
Any company or we can say company's project manager, will not plan the work with loss to the company.
So, optimum time and cost for this numerical will be the initially found values by CPM only.
Here, we consider that optimum duartion is associated with the minimum cost of the project (relating the problem practically).
But, if we want to find optimum duration with compromising the project cost (i.e. cost will increase), then we can go for crashing, the same way explained in the Crashing video, until all the activities get converted to critical one (ignore stopping when cost increased than the last step).
Suggested Notes:
Modified Distribution Method (MODI) | Transportation Problem | Transportation Model
Stepping Stone | Transportation Problem | Transportation Model
Vogel’s Approximation Method (VAM) | Method to Solve Transportation Problem | Transportation Model
Transportation Model - Introduction
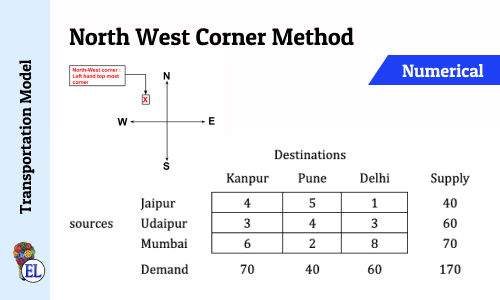
North West Corner Method | Method to Solve Transportation Problem | Transportation Model
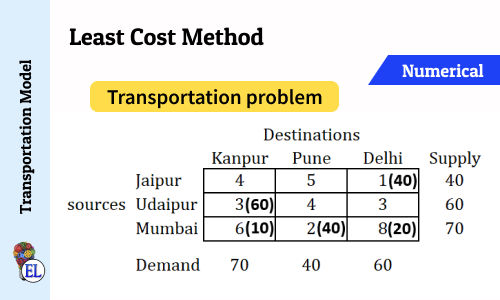
Least Cost Method | Method to Solve Transportation Problem | Transportation Model
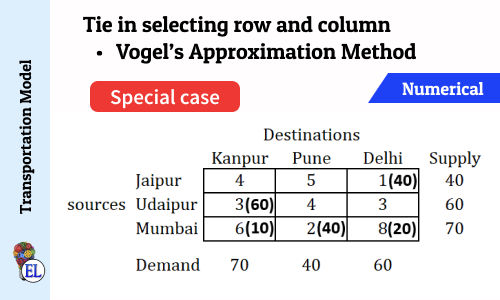
Tie in selecting row and column (Vogel's Approximation Method - VAM) | Numerical | Solving Transportation Problem | Transportation Model
Assignment Model | Linear Programming Problem (LPP) | Introduction
Crashing Special Case - Multiple (Parallel) Critical Paths
Basics of Program Evaluation and Review Technique (PERT)
Numerical on PERT (Program Evaluation and Review Technique)
Network Analysis - Dealing with Network Construction Basics
Construct a project network with predecessor relationship | Operation Research | Numerical
Graphical Method | Methods to solve LPP | Linear Programming
Basics of Linear Programming
Linear Programming Problem (LPP) Formulation with Numericals

Comments:
All comments that you add will await moderation. We'll publish all comments that are topic related, and adhere to our Code of Conduct.
Want to tell us something privately? Contact Us
Post comment