Critically Damped System (ξ = 1)

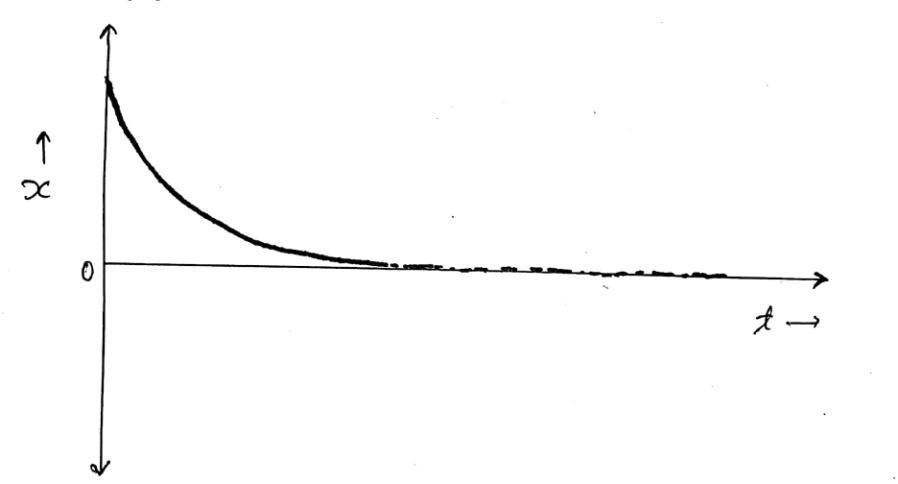Fig.1(Critically Damped System)

• Critically damped system(ξ=1): If the damping factor ξ is equal to one, or the damping coefficient c is equal to critical damping coefficient "c_c", then the system is said to be a critically damped system.

• Two roots for critically damped system are given by S₁ and S₂ as below:

• For $ξ=1$; $S_1 = S_2 = -\omega_n$
  Here both the roots are real and equal, so the solution to the differential equation can be given by

• Now differentiating equation (1) with respect to ‘t’, we get:

• Now, let at
  $t = 0$ : $x = X_0$
  $t = 0$ : $\mathring x =0$

• Substituting these values in equation (1):

• Same way, from equation (2), we get

• Now putting the values of A and B in equation (1), we get:

Conclusion

• From above equation (5), it is seen that as time t increases, the displacement x decreases exponentially.

• The motion of a critically damped system is aperiodic (aperiodic motion motions are those motions in which the motion does not repeat after a regular interval of time i.e non periodic motion) and so the system does not shows vibrations.

• For critically damped systems, if a system is displaced from its initial position, it will try to reach its mean position in a very short time.

• Critically damped systems are generally seen in hydraulic doors closer as it is necessary for the door to come to its initial position in a very short time.